This article is part of the TechXchange: Delving into EMI, EMC and Noise
This file type includes high-resolution graphics and schematics when applicable.
1. Decreasing the resistor values in the circuit always improves noise performance.
It’s a well-known relationship that noise voltage increases with higher resistor values according to the Johnson noise equation:
erms = √(4kTRB)
where erms is the rms voltage noise, k is Boltzmann’s constant, T is temperature in Kelvin, R is the resistance, and B is the bandwidth.
This leads many engineers to the conclusion that resistor values should be shrunk in order to reduce the noise. Although this is often true, it can’t be assumed because specific examples show that larger resistors improve the noise performance.
For instance, in most cases, current is measured by passing it through a resistor and measuring the resulting voltage. The voltage developed is proportional to the resistor value according to Ohm’s law, V=I·R. However, as shown above, the Johnson noise of the resistor is proportional to the square root of the resistor value. Because of this relationship, a 3-dB improvement in the signal-to-noise ratio can be achieved each time the resistor value is doubled. This trend continues right up to the point where the voltage developed is too large or the power dissipated is too high.
2. The noise spectral density of all noise sources can be added up and the bandwidth taken into account at the end of the calculation.
It can save time to combine the noise spectral density (nV/√Hz) of multiple noise sources (voltage noise sources are combined as the root sum of squares) rather than computing the rms noise of each noise source separately. But this simplification is only applicable if the bandwidth seen by each noise source is the same.
It becomes a dangerous trap if the bandwidths seen by each of the noise sources are different. The figure shows the implications in an oversampled system. It would appear from the noise spectral density that the gain amplifier will dominate the total noise of the system. However, once the bandwidth is considered, the rms noise contributed by each stage is very similar.
3. It’s important to include every noise source in hand calculations.
It may be tempting to consider every noise source in a design, but a designer’s time is valuable and such scrutiny can be very time-consuming in large designs. Comprehensive noise calculations are best left to simulation software.
Still, how does a designer simplify the hand noise calculations needed during the design process? Ignore minor noise sources below a certain threshold. If a noise source is 1/5th the rms value of the dominant noise source (or any other noise source referred to the same point), it contributes less than 2% to the total noise and can reasonably be ignored. Designers argue, to some degree, where to draw the threshold line, below which it’s not necessary to consider a noise source. At some level, though, whether it’s 1/3rd, 1/5th, or 1/10th (which add 5%, 2% and 0.5% to the total noise, respectively), it’s not worth worrying about smaller noise sources than that until the design is fixed enough to simulate or calculate fully.
4. Pick an ADC driver with 1/10th the noise of the ADC.
Analog-to-digital converter (ADC) datasheets may suggest driving the analog input with a low-noise ADC driver amplifier that has something like 1/10th the noise of the ADC. However, this isn’t always the best choice. In a system, it’s often worth examining the tradeoff of the ADC driver noise from a system level.
First, if the noise sources in the system preceding the ADC driver are much larger than the ADC driver noise, then choosing a very-low-noise ADC driver will not provide any system benefit. In other words, the ADC driver noise should be commensurate with the rest of the system.
Second, even in the simple case where there’s just an ADC and an amplifier to drive it, it may still be advantageous to examine the noise tradeoff and determine its effects on the system. The reason for this can be made clearer with a numerical example. Consider a system that uses a 16-bit ADC with an SNR value that equates to 100-µV rms noise, and an amplifier with 10-µV rms noise as the ADC driver. The total noise when these sources are combined as the root-sum-of-squares is 100.5 µV rms; very close to the noise of the ADC alone.
The following two options that bring the amplifier and ADC into closer balance can be considered, as well as the effects on system performance. If the 16-bit ADC is replaced with a similar 18-bit ADC that specifies SNR equivalent to 40-µV rms noise, the total noise would change to 41 µV rms. Alternatively, if the 16 bit ADC is retained, but the driver is replaced with a lower-power amplifier that contributes 30-µV rms noise, the total noise would be 104 µV rms. One of these tradeoffs may be a better choice for system performance than the original combination. It’s just a matter of evaluating the tradeoffs and their effects on the overall system.
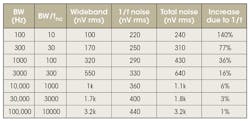
5. 1/f noise must always be considered in dc-coupled circuits.
Because 1/f noise defies many of the usual noise-rejection techniques like low-pass filtering, averaging, and long integrations, it’s a menace to very low-frequency circuits. However, many dc circuits are dominated by white noise sources. In fact, they’re dominated to the point where it’s not useful to calculate the 1/f noise, because it doesn’t add to the total noise.
To see this effect, consider an amplifier with a 1/f noise corner (fnc) at 10 Hz and a wideband noise of 10 nV/√Hz. The noise in a 10-second acquisition is computed for various bandwidths with and without the 1/f noise to determine the effect of leaving it out. In this case, wideband noise begins to dominate when the bandwidth is 100 times fnc, and 1/f noise isn’t significant when the bandwidth is more than 1000 times fnc. Good, modern bipolar amplifiers can have noise corners well below 10 Hz, and zero-drift amplifiers virtually eliminate 1/f noise altogether.
6. Since the 1/f noise increases at lower frequencies, dc circuits have infinite noise.
Although dc is a useful concept for circuit analysis, the truth is that if dc is defined as operation at 0 Hz, then there really is no such thing. As the frequency gets lower and lower, approaching 0 Hz, the period gets longer and longer, approaching infinity. The implication is that a minimum frequency can be seen, even in a circuit that theoretically responds to dc.
This minimum frequency depends on the length of the acquisition, or the aperture time, which is the length of time the device’s output is being watched. If an engineer turns on a device and watches the output for 100 seconds, the lowest-frequency artifact they could observe would be 0.01 Hz. This also means the lowest-frequency noise that can be observed is 0.01 Hz, too.
To extend this with a numerical example, consider a dc-to-1-kHz circuit in which the output is continuously monitored. If a certain amount of 1/f noise is observed in the circuit in the first 100 seconds, from 0.01 Hz to 1 kHz (5 decades of frequency), then the amount of noise observed in 30 years, which is about 1 nHz (12 decades), can be calculated as √(12/5) = 1.55, or 55% more noise than was observed in the first 100 seconds.
This somewhat banal increase even assumes the worst case—1/f noise continues to increase down to 1 nHz, for which there is, so far, no measured evidence. In theory, when the aperture time isn’t well-defined, the 1/f noise could be calculated down to a frequency equal to one over the lifetime of the circuit. In practice, these very long timeline variations are dominated by aging effects and long-term drift rather than 1/f noise. Many engineers set a minimum frequency, such as 0.01 Hz or 1 mHz, for noise calculations in dc circuits to keep the calculations practical.
7. The Noise Equivalent Bandwidth is a multiplier for the noise.
The noise equivalent bandwidth (NEB) is a useful simplification for noise calculations. Some noise from beyond the bandwidth of the circuit can get into the circuit because the gain above the cutoff frequency is not zero. The NEB is the cutoff frequency of a calculated, ideal brick-wall filter that would let in the same amount of noise as would the actual circuit. The NEB is larger than the –3-dB bandwidth and has been calculated for common filter types and orders. For example, it’s 1.57 times larger than the –3-dB bandwidth for a 1-pole low-pass filter, or, in equation form, NEB1pole = 1.57 · BW3dB.
However, there seems to be consistent confusion about where to put that multiplication factor in the noise equation. Remember that the NEB is an adjustment for the bandwidth, not the noise; therefore, it goes under the square root as follows:
eRMS = NSD · √NEB1pole = NSD * √(1.57 · BW3db)
8. The amplifier with the lowest voltage noise is the best choice.
When choosing an op amp, the voltage noise is often the only noise specification considered by the designer. But it’s important not to overlook the current noise as well. Except in special cases such as input-bias-current compensation, the current noise is typically the shot noise of the input bias current:
in = √(2 · q · Ib)
The current noise is converted to a voltage via the source resistance. As a result, when a large resistance is in front of the amplifier input, the current noise can be a larger noise contributor than the voltage noise. Current noise typically becomes a problem when using a low-noise op amp with a large resistance in series with the input.
For example, consider Analog Devices’ ADA4898-1 low-noise op amp with a 10-kΩ resistor in series with the input. The voltage noise of the ADA4898-1 is 0.9 nV/√Hz, the 10-kΩ resistor has 12.8 nV/√Hz, and the 2.4-pA/√Hz current noise times the 10-kΩ resistor is 24 nV/√Hz—the largest noise source in the system. In such cases where the current noise dominates, it’s often possible to find a part with lower current noise and thereby reduce system noise.
This is especially true for precision amplifiers, but high-speed FET-input op amps can help in high-speed circuits as well. For example, instead of choosing the ADA4898-1 and not getting the benefit of the 0.9-nV/√Hz voltage noise, one could choose a JFET input amplifier such as the AD8033 or the ADA4817-1.
9. The best noise performance is achieved by taking lots of gain in the first stage.
It’s often suggested that the gain should be taken in the first stage for better noise performance, which is true because the signal will then be larger relative to the noise of subsequent stages. However, the drawback of taking gain is that it reduces the maximum signal that can be accommodated by the system.
In some cases, rather than taking a large amount of gain in the first stage—which improves the sensitivity of the measurement but limits the dynamic range—it may be better to limit the amount of gain taken in the first stage. Then digitize it with high resolution to maximize both sensitivity and dynamic range.
10. All resistor types have the same noise for a given resistance.
The Johnson noise of resistors is fundamental, giving rise to a simple equation for the noise of a certain resistor at a certain temperature. However, Johnson noise is the least amount of noise that can be observed in a resistor, and it doesn’t mean that all resistor types are created equal with respect to noise.
There’s also excess noise, which is a source of 1/f noise in resistors that’s highly dependent on the resistor type. Excess noise, somewhat confusingly also called current noise, is associated with the way current flows in a discontinuous medium. It’s specified as a noise index (NI) in dB, referred to 1 µV rms/VDC per decade. This means that if there’s 1 V dc across a resistor with a 0 dB NI, the excess noise in a given frequency decade is 1 µV rms.
Carbon- and thick-film resistors have some of the highest NI, ranging up to roughly +10 dB; thus, it’s best to avoid them in noise-sensitive parts of the signal path. Thin films are generally much better at around –20 dB, and metal foil and wirewound resistors can drop below –40 dB.
11. Given enough acquisitions, averaging reduces the noise indefinitely.
Averaging is recognized as a way to reduce the noise by the square root of the number of averages. This is conditionally true when NSD is flat. However, this relationship breaks in the 1/f range as well as in a few other cases.
Consider the case of averaging in a system sampling at a constant frequency (fs), whereby n samples are averaged and decimated by n, and some number m decimated samples are returned. Taking n averages moves the effective sampling rate after decimation to fs/n, reducing the effective maximum frequency seen by the system by a factor of n and reducing the white noise by √n. However, it also took n times longer to obtain m samples, so the lowest frequency that can be seen by the system is also reduced by a factor of n (remember, there’s no such thing as 0 Hz).
The more averages are taken, the lower these maximum and minimum frequencies move on the frequency band. Once the maximum and minimum frequencies are both within the 1/f range, the total noise depends only on the ratio of these frequencies. Therefore, increasing the number of averages provides no further benefit to the noise. The same logic holds for long integration times for an integrating ADC such as multi-slope.
Beyond this mathematical exercise, other practical limits are in play as well. For example, if quantization noise is the dominant noise source, whereby the output of the ADC with a dc input voltage is a constant code with no flicker, then any number of averages will return the same code.
References:
C. D. Motchenbacher, J. A. Connelly (1993). Low-Noise Electronic System Design, Wiley.
"Noise Analysis in Precision Analog Designs" webinar, speaker Scott Hunt, sponsored by Analog Devices and Avnet in conjunction with Electronic Design.
This article is part of the TechXchange: Delving into EMI, EMC and Noise
