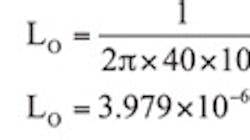Engineers often have trouble analyzing and achieving stability for the linear regulators they employ. Given how little stability information is published by the manufacturers of these devices, this is not surprising. In most linear regulators, the output capacitors’ ESR provides the control loop zero, stabilizing the regulator. The datasheet generally offers little information regarding the stability of the regulator as a function of the load current, output capacitance and output capacitor ESR, which are the external parameters which impact the stability of the regulator. Additionally, the latter two parameters are often uncontrolled by the manufacturer. Past articles on this topic have focused on the criticality and impact of phase margin and overall stability, as well as how to measure the stability using either invasive or non-invasive techniques, therefore, this article provides a different perspective. We discuss a method here to determine the capacitor ESR required to achieve a particular phase margin for any output capacitance value, based on a single simple measurement. That is, if you know the output capacitance and output load current, you can tell what value of ESR will lead to a configuration with the desired phase margin.
Linear Regulator
The majority of linear regulators, regardless of the internal topology, reflect an output impedance that provides all of the information necessary to determine the ESR required to achieve a particular degree of stability.
Without performing an analysis or derivation of the shape of the impedance response, it is possible to measure the output impedance of the voltage regulator without any output capacitors connected to it. Not all regulators are stable in this condition, though most are. The impedance result can be segmented into three distinctive regions. At DC and low frequencies, the output impedance is resistive, with the resistance being related to the load regulation of the regulator and circuit trace resistances. In the second region, the impedance is inductive, with the inductance being dependent on the load current and the bandwidth of the regulator. In the third region, it is possible that the output impedance is again resistive, or not, depending on the regulator.
Since this method is based on the magnitude and phase of the output impedance, the first step to defining the required ESR is to measure the wideband output impedance. We can can easily and inexpensively accomplish this using the OMICRON-Lab Bode 100 VNA and the Picotest J2111A Current Injector as shown in Fig. 1. The selection of these two pieces of test equipment is due to their wide bandwidth and their ability to directly measure both the phase margin and effective Q from the output impedance measurement. The measurement should be made at the lowest expected operating current, since this condition generally results in the poorest phase margin. In fact, the minimum load requirement is often the limit to the achievable performance of the regulator. [1]
Measuring Output Impedance
The output impedance of an LM317 voltage regulator operating at 25mA and at 50mA is shown in Fig. 2. This impedance measurement clearly shows the three regions, as well as confirming that these impedances are dependent on load current. In addition to load current, the output impedance is also affected by the output voltage of the regulator and by the internal compensation of the regulator, so different regulators will yield different results.
The equivalent circuit representing the regulator at 3.3V and 25mA is shown in Fig. 3.
The equivalent circuit inductance is determined by selecting a point in the inductive region. Selecting 1 Ω at 40 kHz at 25mA operating current, Lo can be calculated as:
Next, the values of RS and RP are taken directly from the impedance measurement as 120 mΩ and 10 Ω, respectively. Much of this resistance is from the contact resistance of the connections on the VRTS (Voltage Regulator Test Standard).
The derivation of the ESR requirement is beyond the scope of this article, however, it can be directly calculated as a function of the equivalent parameters, desired output capacitance and desired phase margin (PM).
Where: COUT, RS , RP, and LO are shown in Fig. 3.
PM = Phase margin
Re signifies the real terms of the circuit; the imaginary terms are not included.
The bandwidth of the regulator can be calculated from the equivalent inductance and the output capacitance.
Example
Using the LM317 at an output voltage of 3.3V and an operating load current of 25mA, the values of LO, RP and RS can be determined from Fig. 2. A 22µF capacitor is selected as the output capacitor.
The expected bandwidth of the regulator is calculated from the equivalent inductance and the output capacitance, using the well known resonant frequency relationship for an inductor and capacitor.
Arbitrarily choosing a desired phase margin of 38 degrees, the required ESR is calculated to be 142 mΩ.
A 22µF tantalum capacitor sample is selected and measured, using the OMICRON Lab Bode-100 and B-SMC adapter. A detailed application note describing this method can be found at http://www.omicron-lab.com/application-notes/capacitor-esr-measurement.html. The capacitance and ESR results are shown in Fig. 4.
The capacitance and ESR are both close to the desired values. Finally, using the Bode 100 and J2111A to make a non-invasive phase margin measurement provides the phase margin result at a load current of 25mA. The results, shown in Fig. 5, indicate a phase margin of 38 degrees and a bandwidth of approximately 16 kHz, confirming the mathematical result.
The non-invasive phase margin support, offered by the Bode 100 VNA and J2111A Current Injector allows this method to be used even with fixed voltage regulators, where there is no control loop access. The stability improvements that can be realized in the regulator may enhance many system level performance characteristics, such as output impedance, dynamic step load response, PSRR, reverse transfer and crosstalk.
References
1. “No-Load Specification Impacts Power-Supply Performance,” Steven M. Sandler, Charles Hymowitz, Power Electronics Technology, 2008, Vol. 34 No 3.
2. “Invasive and Non-Invasive Stability Measurements,” https://www.picotest.com/blog/?p=312 2010.
3. Picotest Signal Injector Documentation. Version 1.0c. 2010.
4. “Capacitor ESR Measurement Application Note,” http://www.omicron-lab.com/application-notes/capacitor-esr-measurement.html 2010.
5. Picotest Voltage Regulator Test Standard. Version 1.0d. 2010.
6. “Fundamentals of Power Electronics,” Erickson, Robert W. and Maksimovic, Dragan, Springer, 2004.
Related Articles
SPICE Model Supports LDO Regulator Designs
No-Load Specification Impacts Power-Supply Performance